For example, in an inverter the semiconductors often manipulate 40 times their rated power or more. A small design error, unexpected thermal problem, or minor change in layout could alter this somewhat, perhaps to a factor of 45. This small change puts large additional stresses on the devices, and

can lead to quick failure. The first issue for reliability in power electronic circuits is that of managing device voltage, current, and power dissipation levels to keep them well within rating limits. This can be challenging when power handling levels are high.

The second issue for reliability is simplicity. It is well-established in military electronics that the more parts there are in a system, the more likely it is to fail. Power electronic circuits tend to have few parts, especially in the main energy flow paths. Necessary operations must be carried out through shrewd use of these parts. Often, this means that sophisticated control strategies are applied to seemingly simple conversion circuits.

The third issue for reliability is integration. One way to avoid the reliability-complexity tradeoff is to integrate multiple components and functions on a single substrate. A microprocessor, for example, might contain more than a million gates. As all interconnections and signals flow within a single chip, the reliability is nearly that of a single part. An important parallel trend in power electronic devices involves the integrated module [6]. Manufacturers seek ways to package several switching devices, with their interconnections and protection components together as a unit. Control circuits for converters are also integrated as much as possible to keep reliability high. The package itself becomes a fourth issue for reliability, and one that is as yet only partly understood. Semiconductor packages include small bonding wires that can be susceptible to thermal or vibration damage. The small geometries tend to enhance electromagnetic interference among the internal circuit components.

1.3 Trends in Power Supplies

As costs of electronics decline, the power supply becomes a larger fraction of system cost and design effort. One major manufacturer estimates that power supply cost will soon reach 50% of the total cost of a typical electronic product such as a cordless telephone or personal computer. Thus, new technology developments in power supplies are critically important. In the past, bulky linear power supplies were designed with transformers and rectifiers from the ac line frequency to provide low-level dc voltages for electronic circuits. Late in the 1960s, use of dc sources in aerospace applications led to the development of power electronic dc-dc conversion circuits for power supplies. In a typical power electronics arrangement today, an ac source from a wall outlet is rectified without any transformation; the resulting high dc voltage is converted through a dc-dc circuit to the 5 V, 12 V, or other level required. These switched-mode power supplies are rapidly supplanting linear supplies across the full spectrum of circuit applications.

A personal computer commonly requires three different 5 V supplies, two +12 V supplies, a -12 V supply, a 24 V supply, and perhaps a few more. This does not include supplies for

video display or peripheral devices. Only a switched-mode supply can support such complex requirements without high costs. The bulk and weight of linear supplies make them infeasible for hand-held communication devices, calculators, notebook computers, and similar equipment. Switched-mode supphes often take advantage of MOSFET semiconductor technology. Trends toward high reliability, low cost, and miniaturization have reached the point at which a 5 V power supply sold today might last 1,000,000 hr (more than 100 yr), provide 100 W of output in a package with volume < 15 cm, and sell for a price of < $0.30 watt. This type of supply brings an interesting dilemma: the ac line cord to plug it in actually takes up more space than the power supply itself. Innovative concepts such as integrating a power supply within a connection cable will be used in the future.

Device technology for power supphes is being driven by expanding needs in the automotive and telecommunications industries as well as in markets for portable equipment. The automotive industry is making a transition to 42 V systems to handle increasing electric power needs. Power conversion for this industry must be cost effective, yet rugged enough to survive the high vibration and wide temperature range to which a passenger car is exposed. Global communication is possible only when sophisticated equipment can be used almost anywhere. This brings a special challenge, because electrical supphes are neither reliable nor consistent throughout much of the world. While in North America voltage swings in the domestic ac supply are often < ±5% around a nominal value, in many developing nations the swing can be ±25%-when power is available. Power converters for communications equipment must tolerate these swings, and must also be able to make use of a wide range of possible backup sources. Given the enormous size of worldwide markets for telephones and consumer electronics, there is a clear need for flexible-source equipment. Designers are challenged to obtain maximum performance from small batteries, and to create equipment with minimal energy requirements.

1.4 Conversion Examples 1.4.1 Single-Switch Circuits

Electrical energy sources can come in the form of dc voltage sources at various values, sinusoidal ac sources, polyphase sources, and many others. A power electronic circuit might be asked to transfer energy between two different dc voltage levels, between an ac source and a dc load, or between sources at different frequencies. It might be used to adjust an output voltage or power level, drive a nonlinear load, or control a load current. In this section, we consider a few basic converter arrangements and discuss energy conservation as a tool for analysis.

Example 1.3. Consider the circuit shown in Fig. 1.4. It contains an ac source, a switch, and a resistive load. It is a simple but complete power electronic system. Let us assign a (somewhat arbitrary) control scheme to the switch. What if the switch is turned on whenever V > 0, and turned off otherwise? The input and output voltage waveforms are shown in Fig. 1.5. The input has a time average of 0, and root mean square (RMS) value equal to Vpeak/V where V is the maximum value of V. The output has a nonzero average value given by:

(out(O) =

.71/2 -7Г/2

VpeakCOS

37Г/2

= = 0.3183Уреак

(1.1)

and an RMS value equal to V/l. Since the output has nonzero dc voltage content, the circuit can be used as an ac-dc converter. To make it more useful, a lowpass filter would be added between the output and the load to smooth out the ac portion. This filter needs to be lossless, and will be constructed from only inductors and capacitors.

The circuit in the preceding Example acts as a half-wave rectifier with a resistive load. With the hypothesized switch action, a diode can be substituted for the ideal switch. The example confirms that a simple switching circuit can perform power conversion functions. However, notice that a diode is not, in general, the same as an ideal switch. A diode places restrictions on the current direction, while a true switch would not. An ideal switch allows control over whether it is on or off, while a diodes operation is constrained by circuit variables.

Consider a second half-wave circuit, now with a series L-R load, as shown in Fig. 1.6.

Example 1.4. A series diode-L-i circuit has ac voltage-source input. This circuit operates much differently than the half-wave rectifier with resistive load. A diode will be on if forward biased, and off if reverse biased. In this circuit, an off diode will give a current of zero. When the diode is on, the circuit is the ac source with L-R load. Let

figure 1.4 A simple power electronic system. (From Reference [2], copyright © 1998, Oxford University Press, Inc.; used by permission.)

1440

figure 1.5 Input and output waveforms for Exampl

ie 1.4.

0+

figure 1.6 Half-wave rectifier with l-r load for Example 1.5.

the ac voltage be Vq cos(cot). From Kirchhoffs voltage law (KVL),

VQCOs((jot) = L-\- Ri at

(1.2)

Let us assume that the diode is initially off (this assumption is arbitrary, and we will check it out as the example is solved). If the diode is off, the diode current i = 0, and the voltage across the diode will be v. The diode will become forward-biased when v becomes positive. The diode will turn on when the input voltage makes a zero-crossing in the positive direction. This allows us to establish initial conditions for the circuit: {(to) = 0, to = -я/(2ш). The differential equation can be solved in a conventional way to give

i(t) = Уо +

cos(a;t) +

-sin(a;t)

(1.3)

where т is the time constant L/R. What about diode turn-off? One first guess might be that the diode turns off when the voltage becomes negative, but this is not correct. We notice from the solution that the current is

not zero when the voltage first becomes negative. If the switch attempts to turn off, it must drop the inductor current to zero instantly. The derivative of current in the inductor di/dt would become negative infinite. The inductor voltage L(di/dt) similarly becomes negative infinite - and the devices are destroyed. What really happens is that the falling current allows the inductor to maintain forward bias on the diode. The diode will turn off only when the current reaches zero. A diode has definite properties that determine circuit action, and both voltage and current are relevant. Figure 1.7 shows the input and output waveforms for a time constant т equal to 1/3 of the ac waveform period.

1.4.2 The Method of Energy Balance

Any circuit must satisfy conservation of energy. In a lossless power electronic circuit, energy is delivered from source to load, which is possible through an intermediate storage step. The energy flow must balance over time such that the energy drawn from the source matches that delivered to the load. The converter in Fig. 1.8 serves as an example of how the method of energy balance can be used to analyze circuit operation.

Example 1.5. The switches in the circuit of Fig. 1.8 are controlled cyclically to operate in alternation: when the left switch is on, the right one is off, and so on. What does the circuit do if each switch operates half the time? The inductor and capacitor have large values.

When the left switch is on, the source voltage V appears across the inductor. When the right switch is on, the output voltage V appears across the inductor. If this circuit is to be a useful converter, we want the inductor to receive energy from the source, then deliver it to the load without loss. Over time, this means that energy does not build up in the inductor (instead it flows through on average). The power into the inductor therefore must equal the power out, at least over a cycle. Therefore, the average power in should equal the average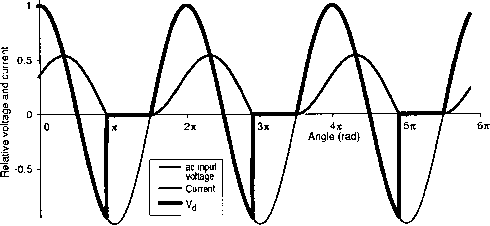

-1 ->

figure 1.7 Input and output waveforms for Example 1.5.

figure 1.8 Energy transfer switching circuit for Example 1.5. (From Reference [2], copyright © 1998, Oxford University Press, Inc.; used by permission.)

power out of the inductor. Let us denote the inductor current as i. The input is a constant voltage source. Because L is large, this constant voltage source will not be able to change the inductor current quickly, and we can assume that the inductor current is also constant. The average power into L over the cycle period T is

p. -1

in rj.

(1.4)

The method of energy balance shows that when operated as described in the example, the circuit of Fig. 1.8 serves as a polarity reverser. The output voltage magnitude is the same as that of the input, but the output polarity is negative with respect to the reference node. The circuit is often used to generate a negative supply for analog circuits from a single positive input level. Other output voltage magnitudes can be achieved at the output if the switches alternate at unequal times.

If the inductor in the polarity reversal circuit is moved instead to the input, a step-up function is obtained. Consider the circuit of Fig. 1.9 in the following example.

Example 1.6. The switches in Fig. 1.9 are controlled cychcally in alternation. The left switch is on for 2/3 of each cycle, and the right switch for 1/3 of each cycle. Determine the relationship between V and V.

The inductors energy should not build up when the circuit is operating normally as a converter. A power balance calculation can be used to relate the input and output voltages. Again let i be the inductor current.

For the average power out of L, we must be careful about current directions. The current out of the inductor will have a value -i. The average output power is

P -i

out Y

dt = -

(1.5)

For this circuit to be useful as a converter, there is net energy flow from the source to the load over time. The power conservation relationship P = P requires that

figure 1.9 Switching converter Example 1.6. (From Reference [2], copyright © 1998, Oxford University Press, Inc.; used by permission.)

When the left switch is on, power is injected into the inductor. Its average value is

- (1.6)

P- = -

v-j dt =

Power leaves the inductor when the right switch is on. Again we need to be careful of polarities, and remember that the current should be set to negative to represent output power. The result is

p -1

out Y

-(Vi-V,Jidt = - +

When the input and output power are equated,

V:J K,

= - + -

and 3V: =

(1.7)

(1.8)

and we see that the output voltage is triple the input. Many seasoned engineers find the dc-dc step-up function of Fig. 1.9 to be surprising. Yet Fig. 1.9 is just one example of such an action. Others (including flyback circuits related to Fig. 1.8) are used in systems from CRT electron guns to spark ignitions for automobiles.

All the circuits in the preceding examples have few components, provide useful conversion functions, and are efficient. If the switching devices are ideal, each circuit is lossless. Over the history of power electronics, development has tended to flow around the discovery of such circuits, that is, a circuit with a particular conversion function is discovered, analyzed, and apphed. As the circuit moves from simple laboratory testing to a complete commercial product, control and protection functions are added. The power portion of the circuit remains close to the original idea. The natural question arises as to whether a systematic approach to conversion is possible. Can we start with a desired function and design an appropriate converter, rather than starting from the converter and working backwards toward the application? What underlying principles can be applied to design and analysis? In this introductory chapter, we will introduce a few of the key concepts. Keep in mind that while many of the circuits look deceptively simple, all are nonlinear systems with unusual behavior.

1.5 Tools For Analysis and Design 1.5.1 The Switch Matrix

The most readily apparent difference between a power electronic circuit and other types of electronic circuits is the switch action. In contrast to a digital circuit, the switches do not indicate a logic level. Control is effected by determining the times at which switches should operate. Whether there is just one switch or a large group, there is a complexity limit: If a converter has m inputs and n outputs, even the densest

possible collection of switches would have a single switch between each input Ипе and each output line. The m x n switches in the circuit can be arranged according to their connections. The pattern suggests a matrix, as shown in Fig. 1.10.

Power electronic circuits fall into two broad classes:

1. Direct switch matrix circuits. In these circuits, energy storage elements are connected to the matrix only at the input and output terminals. The storage elements effectively become part of the source or load. A rectifier with an external lowpass filter is an example of a direct switch matrix circuit. In the literature, these circuits are sometimes called matrix converters.

2. Indirect switch matrix circuits, also termed embedded converters. These circuits, like the polarity-reverser example, have energy storage elements connected within the matrix structure. There are usually very few storage elements. Indirect switch matrix circuits are most commonly analyzed as a cascade connection of direct switch matrix circuits with the storage in between.

The switch matrices in realistic applications are small. A 2 x 2 switch matrix, for example, covers all possible cases with a single-port input source and a two-terminal load. The matrix is commonly drawn as the H-bridge shown in Fig. 1.11. A more complicated example is the three-phase bridge rectifier shown in Fig. 1.12. There are three possible inputs, and the two terminals of the dc circuit provide outputs, which give a 3x2 switch matrix. In a personal computer power supply, there are commonly five separate dc loads, and the switch matrix is 2 x 10. Very few practical converters have more than 24 switches, and most designs use fewer than 12.

A switch matrix provides a way to organize devices for a given application. It also helps to focus the effort into three major task areas. Each of these areas must be addressed

m input lines

т X п switches

п output lines

figure 1.10 The general switch matrix.

Input Source

Ъ-\\

I--Load--1

,2 2,2

figure 1.11 H-bridge configuration of a 2 x 2 switch matrix..

Dc load

figure 1.12 Three-phase bridge rectifier circuit, a 3x2 switch matrix.

effectively in order to produce a useful power electronic system.

The Hardware Task Build a switch matrix. This involves the selection of appropriate semiconductor switches and the auxiliary elements that drive and protect them.

The Software Task Operate the matrix to achieve the desired conversion. All operational decisions are implemented by adjusting switch timing.

The Interface TaskAdd energy storage elements to provide the filters or intermediate storage necessary to meet the apphcation requirements. Unlike most filter applications, lossless filters with simple structures are required.

In a rectifier or other converter, we must choose the electronic parts, how to operate them, and how best to filter the output to satisfy the needs of the load.

1.5.2 Implications of Kirchhoffs Voltage and Current Laws

A major challenge of switch circuits is their capacity to violate circuit laws. Consider first the simple circuits of

Fig. 1.13. The circuit of Fig. 1.13a is something we might try for ac-dc conversion. This circuit has problems. Kirchhoffs voltage law (KVL) tells us that the sum of voltage drops around a closed loop is zero. However, with the switch closed, the sum of voltages around the loop is not zero. In reality, this is not a valid result. Instead, a very large current will flow and cause a large / R drop in the wires. The KVL will be satisfied by the wire voltage drop, but a fire or, better yet, fuse action, might result. There is, however, nothing that would prevent an operator from trying to close the switch. The KVL, then, implies a crucial restriction: A switch matrix must not attempt to interconnect unequal voltage sources directly. Notice that a wire, or dead short, can be thought of as a voltage source with У = 0, so KVL is a generalization for avoiding shorts across an individual voltage source.

A similar constraint holds for Kirchhoffs current law (KCL). The KCL states that currents into a node must sum to zero. When current sources are present in a converter, we must avoid any attempts to violate KCL. In Fig. 1.13b, if the current sources are different and the switch is opened, the sum of the currents into the node will not be zero. In a real circuit, high voltages will build up and cause an arc to create another current path. This situation has real potential for damage, and a fuse will not help. The KCL implies a restriction in which a switch matrix must not attempt to interconnect unequal current sources directly. An open circuit can be thought of as a current source with / = 0, so KCL applies to the problem of opening an individual current source.

In contrast to conventional circuits, in which KVL and KCL are automatically satisfied, switches do not know KVL or KCL. If a designer forgets to check, and accidentally shorts two voltages or breaks a current source connection, some problem or damage will result. On the other hand, KVL and KCL place necessary constraints on the operating strategy of a switch matrix. In the case of voltage sources, switches must not act to create short-circuit paths among dissimilar sources. In the case of KCL, switches must act to provide a path for currents. These constraints drastically reduce the number of valid switch operating conditions in a switch matrix, and lead to manageable operating design problems.

When energy storage is included, there are interesting implications for the current law restrictions. Figure 1.14 shows two circuit law problems. In Fig. 1.14a, the voltage source will cause the inductor current to ramp up indefinitely because У = L di/dt. We might consider this to be a KVL problem, since the long-term effect is similar to shorting the source. In Fig. 1.14b, the current source will cause the capacitor voltage to ramp toward infinity. This causes a KCL problem ; eventually, an arc will form to create an additional current path, just as if the current source had been opened. Of course, these connections are not problematic if they are only temporary. However, it should be evident that an inductor will not support dc voltage, and a capacitor will not support dc current. On average over an extended time interval.

Switch must remain open

Switch must remain closed

figure 1.13 Hypothjetical power converters: (a) Possible ac-dc converter; (b) Possible dc-dc converter. (From [2], copyright © 1998, Oxford University Press Inc., used by permission.)

figure 1.14 Short-term KVL and KCL problems in energy storage circuits: (a) An inductor cannot sustain dc voltage indefinitely; (b) A capacitor cannot sustain dc current indefinitely.

the voltage across an inductor must be zero, and the current into a capacitor must be zero.

1.5.3 Resolving the Hardware Problem: Semiconductor Devices

A switch is either on or off. An ideal switch, when on, will carry any current in any direction. When off, it will never carry current, no matter what voltage is applied. It is entirely lossless, and changes from its on state to its off state instantaneously. A real switch can only approximate an ideal switch. Those aspects of real switches that differ from the ideal include the following:

hmits on the amount and direction of on-state current;

a nonzero on-state voltage drop (such as a diode forward voltage);

some level of leakage current when the device is supposed to be off;

limitations on the voltage that can be applied when off; and

operating speed. The time of transition between the on and off states can be important.

The degree to which properties of an ideal switch must be met by a real switch depends on the application. For example, a diode can easily be used to conduct dc current; the fact that it conducts only in one direction is often an advantage, not a weakness.

Many different types of semiconductors have been applied in power electronics. In general, these fall into three groups:

Diodes, which are used in rectifiers, dc-dc converters, and in supporting roles.

Transistors, which in general are suitable for control of single-polarity circuits. Several types of transistors are applied to power converters. The most recent type, the insulated gate bipolar transistor (IGBT) is unique to power electronics and has good characteristics for applications such as inverters.

Thyristors, which are multijunction semiconductor devices with latching behavior. Thyristors in general can be switched with short pulses, and then maintain their state until current is removed. They act only as switches. The characteristics are especially well-suited to controllable rectifiers, although thyristors have been applied to all power conversion applications.

Some of the features of the most common power semiconductors are listed in Table 1.1. This table shows a wide variety of speeds and rating levels. As a rule, faster speeds apply to lower ratings. For each device type, cost tends to increase both for faster devices and for devices with higher power-handling capacity.

Conducting direction and blocking behavior are fundamentally tied to the device type, and these basic characteristics constrain the choice of device for a given conversion function. Consider again a diode. It carries current in only one direction and always blocks current in the other. Ideally, the diode exhibits no forward voltage drop or off-state leakage current. Although it lacks all the features of an ideal switch, the ideal diode is an important switching device. Other real devices operate with polarity limits on current and voltage and have corresponding ideal counterparts. It is convenient to define a special type of switch to represent this behavior: the restricted switch.

DEFINITION: A restricted switch is an ideal switch with the addition of restrictions on the direction of current

table 1.1 Some modern semiconductor switch types and their basic characteristics

DEVICE TYPE CHARACTERISTICS OF POWER DEVICES

Diode Current ratings from < 1 to > 5000 A. Voltage ratings from 10 V to lOkV or more. The fastest power devices switch in <20ns, while the slowest require 100 is or more. The function of a diode applies in rectifiers and dc-dc circuits.

BJT (Bipolar Junction Transistor) Conducts collector current (in one

direction) when sufficient base current is applied. Power device current ratings from 0.5 to 500 A or more; voltages from 30 to 1200 V Switching times from 0.5 to 100 is. The function applies to dc-dc circuits; combinations with diodes are used in inverters. Power BJTs are being supplanted by FETs and IGBTs.

FET (Field Effect Transistor) Conducts drain current when sufficient gate voltage is applied. Power FETs (nearly always enhancement-mode MOSFETs) have a parallel connected reverse diode by virtue of their construction. Ratings from ~ 1 to ~ 100 A and 30 up to 1000 V Switching times are fast, from 50 or less up to 200 ns. The function applies to dc-dc conversion, where the FET is in wide use, and to inverters.

IGBT (Insulated Gate Bipolar Transistor) A special type of power FET that has the function of a BJT with its base driven by a FET. Faster than a BJT of similar ratings, and easy to use. Ratings from 10 to >600A, with voltages of 600 to 1700 V The IGBT is popular in inverters from ~1 to 100 kW or more. It is found almost exclusively in power electronics applications.

SCR (Silicon Controlled Rectifier) A thyristor that conducts like a diode after a gate pulse is applied. Turns off only when current becomes zero. Prevents current flow until a pulse appears. Ratings from 10 up to more than 5000 A, and from 200 V up to 6kV Switching requires 1 to 200 is. Widely used for controlled rectifiers. The SCR is found almost exclusively in power electronics applications, and is the most common member of the thyristor family.

СТО (Gate Turn-Off Thyristor) An SCR that can be turned off by sending a negative pulse to its gate terminal. Can substitute for BJTs in applications where power ratings must be very high. The ratings approach those of SCRs, and the speeds are similar as well. Used in inverters rated > 100 kW.

TRIAC A semiconductor constructed to resemble two SCRs connected in reverse parallel. Ratings from 2 to 50 A and 200 to 800 V Used in lamp dimmers, home appliances, and hand tools. Not as rugged as many other device types, but very convenient for many ac applications.

MCT (MOSFET Controlled Thyristor) A special type of SCR that has the function of a СТО with its gate driven from a FET. Much faster than conventional GTOs, and easier to use. These devices are supplanting GTOs in some application areas.

flow and voltage polarity. The ideal diode is one example of a restricted switch.

The diode always permits current flow in one direction, while blocking flow in the other. It therefore represents a forward-conducting reverse-blocking restricted switch, and operates in one quadrant on a graph of device current vs voltage. This FCRB function is automatic - the two diode terminals provide all the necessary information for switch action. Other restricted switches require a third gate terminal to determine their state. Consider the polarity possibilities

given in Table 1.2. Additional functions such as bidirectional-conducting reverse-blocking can be obtained simply by reverse connection of one of the five types in the table.

The quadrant operation shown in the table indicates polarities. For example, the current in a diode will be positive when on and the voltage will be negative when off. This means diode operation is restricted to the single quadrant comprising the upper vertical (current) axis and the left horizontal (voltage) axis. The other combinations appear in the table. Symbols for restricted switches can be built up by interpreting the diodes triangle as the current-carrying direction and the bar as the blocking direction. The five types can be drawn as in Table 1.2. Although the symbols are used infrequently, they are valuable for showing the polarity behavior of switching devices. A circuit drawn with restricted switches represents an idealized power converter.

Restricted switch concepts guide the selection of devices. For example, consider an inverter intended to deliver ac load current from a dc voltage source. A switch matrix built to perform this function must be able to manipulate ac current and dc voltage. Regardless of the physical arrangement of the matrix, we would expect bidirectional-conducting forward-blocking switches to be useful for this conversion. This is a correct result: Modern inverters operating from dc voltage sources are built with FETs or with IGBTs arranged with reverse-parallel diodes. As new power devices are introduced to the market, it is straightforward to determine what types of converters will use them.

1.5.4 Resolving the Software Problem: Switching Functions

The physical m x n switch matrix can be associated with a mathematical mx n switch state matrix. Each element of this matrix, called a switching function, shows whether the corresponding physical device is on or off.

DEFINITION: A switching function q(t) has a value of unity when the corresponding physical switch is on and 0 when it is off. Switching functions are discrete-valued functions of time, and control of switching devices can be represented with them.

Figure 1.15 shows a typical switching function. It is periodic, with period T, representing the most likely repetitive switch action in a power converter. For convenience, it is drawn on a relative time scale that begins at 0 and draws out the square wave period-by-period. The actual timing is arbitrary, so the center of the first pulse is defined as a specified time in the figure. In many converters, the switching function is generated as an actual control voltage signal that might drive the gate of either a MOSFET or some other semiconductor switching device.

The timing of switch action is the only alternative for control of a power converter. As switch action can be repre-

Action

Device

Quadrants

Restricted Switch Symbol

Device Symbol

Carries current in one direction, blocks in the other (forward-conducting reverse-blocking)

Carries or blocks current in one direction (forward-conducting, forward-blocking)

Carries in one direction or blocks in both directions (forward-conducting, bidirectional-blocking)

Carries in both directions, but blocks only in one direction (bidirectional-carrying, forward-blocking)

Fully bidirectional

Diode

СТО

Ideal switch

sented with a discrete-valued switching function, the timing can be represented within the switching function framework. Based on Fig. 1.15, a generic switching function can be characterized completely with three parameters:

1. The duty ratio D is the fraction of time during which the switch is on. For control purposes the pulse width can be adjusted to achieve a desired result. We can term this adjustment process pulse-width modulation (PWM), perhaps the most important process for implementing control in power converters.

2. The frequency itch = 1/T (with radian frequency co = 2nf) is most often constant, although not in all applications. For control purposes, frequency can be adjusted. This is unusual in power converters because

the operating frequencies are often dictated by the apphcation.

3. The time delay or phase ф^ = cot. Rectifiers often make use of phase control to provide a range of adjustment. A few speciahzed ac-ac converter applications use phase modulation.

With just three parameters to vary, there are relatively few possible ways to control any power electronic circuit. The dc-dc converters usually rely on duty ratio adjustment (PWM) to alter their behavior. Phase control is common in controlled rectifier applications. Pulse-width modulation is used formally for many types of inverters.

Switching functions are very powerful tools for general representation of converter action [7]. The most widely used

Absolute time reference

DT T T+DT 21 31

Relative Time ~ Period T

figure 1.15 A generic switching function with period T, durty ratio D, and time reference t.

table 1.2 The types of restricted switches

control approaches derive from averages of switching functions [2, 8]. Their utility comes from their application in writing circuit equations. For example, in the boost converter of Fig. 1.9, the loop and node equations change depending on which switch is acting at a given moment. The two possible circuit configurations each have distinct equations. Switching functions allow them to be combined. By assigning switching functions qi(t) and 2(0 to the left and right switching devices, respectively, we obtain

= °) +1 = °) °

42 (У-ш - = l>c), ?2 + = , right switch on

(1.9)

Because the switches alternate, and the switching functions must be 0 or 1, these sets of equations can be combined to give

The combined expressions are simpler and easier to analyze than the original equations.

For control purposes, the average of equations such as (1.10) often proceeds with the replacement of switching functions q with duty ratios d. The discrete time action of a switching function thus will be represented by an average duty cycle parameter. Switching functions, the advantages gained by averaging, and control approaches such as PWM are discussed at length in several chapters in this handbook.

1.5.5 Resolving the Interface Problem: Lossless Filter Design

Lossless filters for power electronics applications are sometimes called smoothing filters [9]. In apphcations in which dc outputs are of interest, such filters are commonly implemented as simple lowpass LC structures. The analysis is facilitated because in most cases the residual output waveform, termed ripple, has a known shape. Filter design for rectifiers or dc-dc converters is a question of choosing storage elements large enough to keep ripple low, but not so large that the whole circuit becomes unwieldy or expensive.

Filter design is more challenging when ac outputs are desired. In some cases, this is again an issue of lowpass filter design. In many apphcations, lowpass filters are not adequate to meet low noise requirements. In this situation, active filters can be used. In power electronics, the term active filter refers to lossless switching converters that actively inject or remove energy moment-by-moment to compensate for distortion. The circuits (discussed elsewhere in this handbook) are not

related to the linear active filter op-amp circuits used in analog signal processing. In ac cases, there is a continuing opportunity for innovation in filter design.

1.6 Summary

Power electronics is the study of electronic circuits for the control and conversion of electrical energy. The technology is a critical part of our energy infrastructure, and supports almost all important electrical applications. For power electronics design, we consider only those circuits and devices that, in principle, introduce no loss and can achieve near-perfect reliability. The two key characteristics of high efficiency and high reliability are implemented with switching circuits, supplemented with energy storage. Switching circuits in turn can be organized as switch matrices. This facilitates their analysis and design.

In a power electronic system, the three primary challenges are the hardware problem of implementing a switching matrix, the software problem of deciding how to operate that matrix, and the interface problem of removing unwanted distortion and providing the user with the desired clean power source. The hardware is implemented with a few special types of power semiconductors. These include several types of transistors, especially MOSFETs and IGBTs, and several types of thyristors, especially SCRs and GTOs. The software problem can be represented in terms of switching functions. The frequency, duty ratio, and phase of the switching functions are available for operational purposes. The interface problem is addressed by means of lossless filter circuits. Most often, these are lossless LC passive filters to smooth out ripple or reduce harmonics. More recently, active filter circuits have been apphed to make dynamic corrections in power conversion waveforms.

Improvements in devices and advances in control concepts have led to steady improvements in power electronic circuits and systems. This is driving tremendous expansion of their application. Personal computers, for example, would be unwieldy and inefficient without power electronic dc supphes. Portable communication devices and computers would be impractical. High-performance hghting systems, motor controls, and a wide range of industrial controls depend on power electronics. In the near future, we can expect strong growth in automotive applications, in dc power supphes for communication systems, in portable apphcations, and in high-end converters for advanced microprocessors. During the next generation, we will reach a time when almost all electrical energy is processed through power electronics somewhere in the path from generation to end use.

References

1. J. Motto, Ed., Introduction to Solid State Power Electronics, Westing-house, Youngwood, PA, 1977.